
Painlevé VI has a family of elliptic solutions and a lot of algebraic solutions with polynomial degree going up to $72$ and genus up to $7$.Ĭoncerning physics applications, one could look at the long list on p.19 here to get an idea. Such solutions include classical special function solutions (for example, for Painlevé II they are expressed in terms of Airy functions, and for Painlevé VI in terms of Gauss hypergeometric functions). You can check, for instance, NIST Library for explicit examples. However, many particular solutions are known for special values of parameters and integration constants. Spring Solved Problems for Family of Curves Differential EquationsLesson 2 - Solving Elementary Differential Equations Elementary Differential Equations. Let x f ( t, x) have the initial condition. Thus, a first order, linear, initial-value problem will have a unique solution. It is known (proven rigorously) that the general solutions of Painlevé equations, in a sense, cannot be expressed in terms of classical functions. The following theorem tells us that solutions to first-order differential equations exist and are unique under certain reasonable conditions.
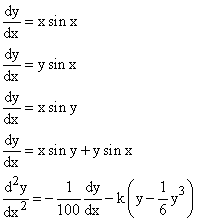
Painlevé equations have a lot of applications in various areas of mathematics, including integrable models, random matrices, algebraic and differential geometry and combinatorics. Differential equations and mathematical modeling can be used to study a wide range of social issues. Any particular solution can be obtained by specifying the arbitrary constants C1 and C2 For example, the particular. Modeling situations with differential equations. This means their solution is a function Learn more in this video.
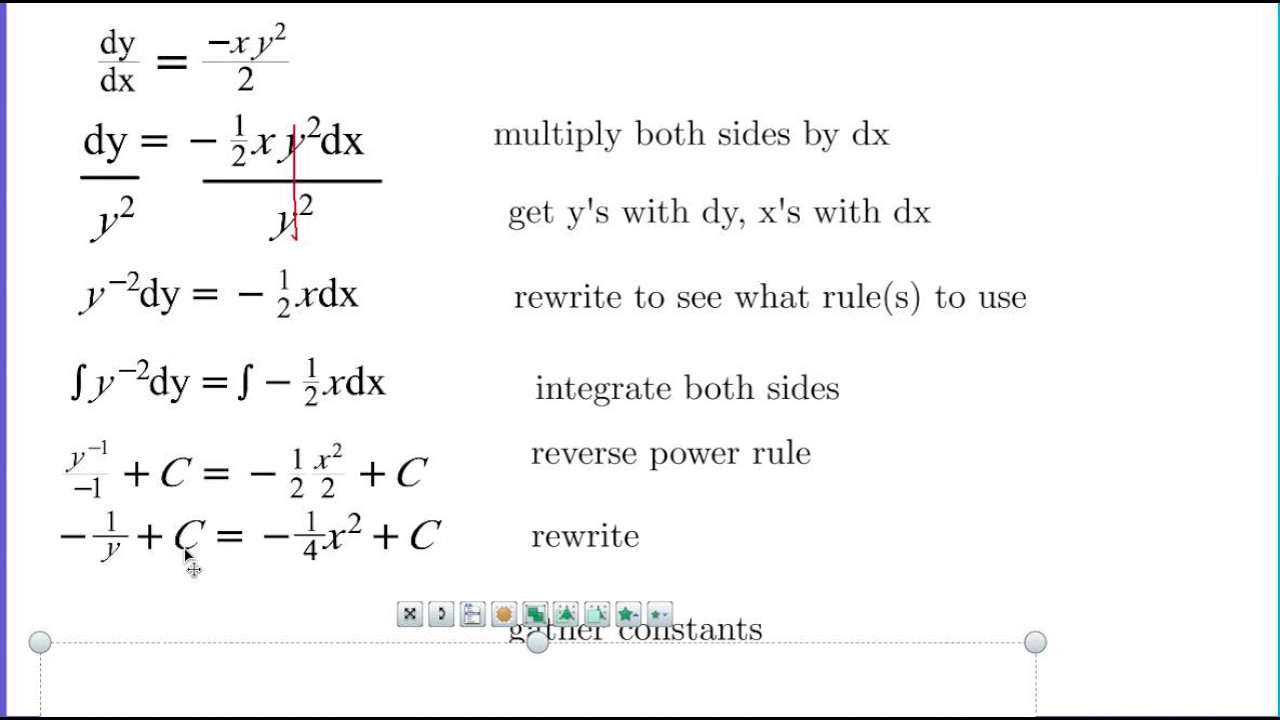
When depends explicitly on the independent time variable, the differential equation. Differential equations are equations that relate a function with one or more of its derivatives. These are the only nonlinear 2nd order ODEs of the form $w''=F(t,w,w')$ whose solutions cannot have movable critical points. Equation () is an example of an autonomous differential equation since.
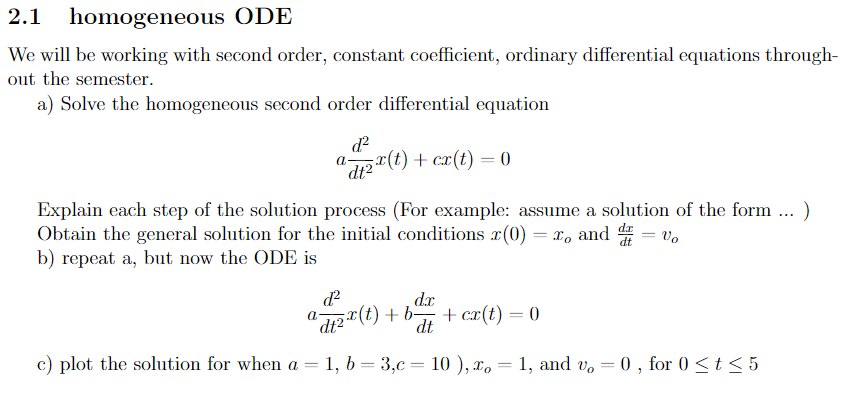
Example A.3 Solve the partial differential. I would certainly like to mention six Painlevé equations. In solving such problems we can make use of the solutions to ordinary differential equations considered earlier.


 0 kommentar(er)
0 kommentar(er)
